Quadratic Equation Solver

Report a Bug

How to use this Quadratic Equation Solver?

1. Enter Coefficients:
   • Input ‘a’: Enter the coefficient a of the quadratic term x² in the first input box.
   • Input ‘b’: Enter the coefficient b of the linear term x in the second input box.
   • Input ‘c’: Enter the constant term c in the third input box.
2. Hover for Info (Optional): Hover over the information icon i next to the equation form to view a tooltip that provides a definition of what a quadratic equation is.
3. Click Solve: After entering the coefficients a, b, and c, click the “Solve” button. The tool will then display the roots of the equation, if they exist.
4. View Results: Below the buttons, the output will be displayed, which may include one or two real roots, or a message indicating that no real roots exist.
5. Reset the Tool (Optional): If you wish to solve another equation, click the “Reset” button to clear all the input fields and the output.

Understanding Quadratic Equations

Introduction

Quadratic equations are a cornerstone of algebra and the foundation for polynomial equations of higher degrees. They serve as a simple yet powerful tool in various areas of mathematics, physics, engineering, and finance. This blog aims to demystify the quadratic equation, break down its components, and provide practical applications.

What is a Quadratic Equation?

Definition

A quadratic equation is a second-order polynomial equation that can be represented in the standard form:

ax² + bx + c = 0

Here, a, b, and c are real numbers, and a is not equal to zero.

Components of a Quadratic Equation

• ax²: Quadratic term
• bx: Linear term
• c: Constant term

Solving Quadratic Equations

There are multiple methods to solve a quadratic equation:

Factoring

Factoring involves expressing the equation as a product of its roots.

Quadratic Formula

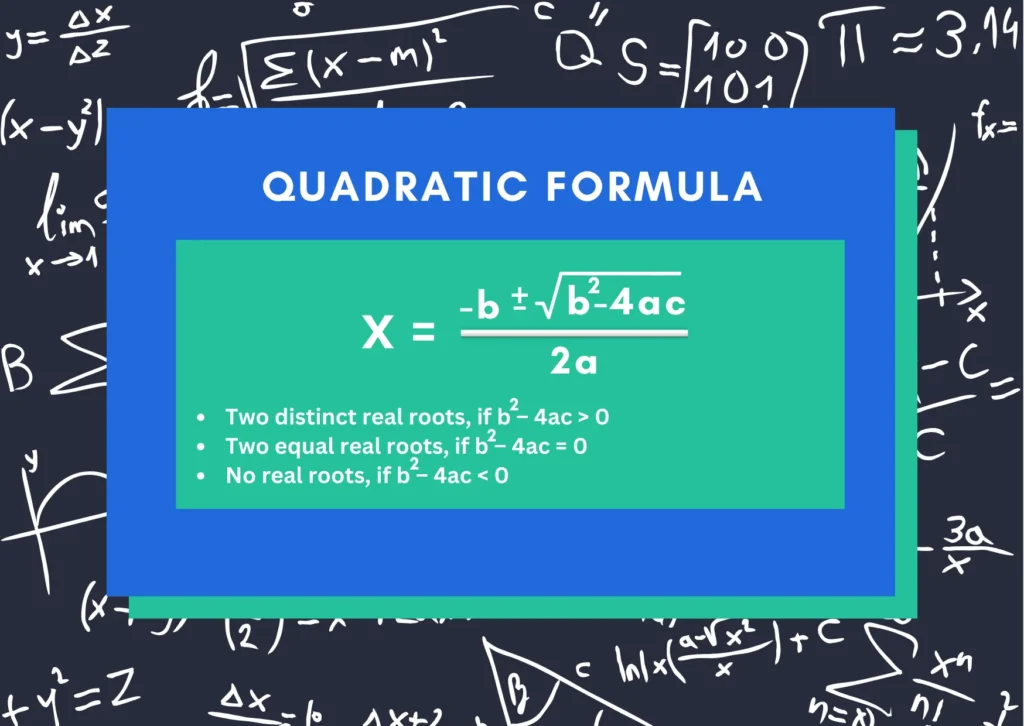
This quadratic formula is a straightforward method to find the roots directly.

Completing the Square

Completing the square is a more laborious technique but can be useful for understanding the geometrical interpretation of the equation.

Applications of Quadratic Equations

1. Physics
   • Projectile Motion: The equations of motion for projectiles are often quadratic.
   • Optics: Quadratic equations can describe the paths of rays of light in various media.
   • Harmonic Motion: The equations for certain types of oscillations and waves are quadratic.
2. Engineering
   • Civil Engineering: In load calculations for building structures.
   • Mechanical Engineering: In problems related to velocity and acceleration.
   • Electrical Engineering: In analyzing circuits and electrical networks.
3. Finance
   • Investment Compounding: Quadratic equations can model certain types of interest rate growth.
   • Optimization Problems: Such as maximizing profit given certain constraints.
4. Computer Science
   • Algorithms: Quadratic equations can model the time complexity of certain algorithms.
   • Graphics: Quadratic equations can be used in rendering curves and calculating distances in computer graphics.
5. Medicine
   • Pharmacology: In modeling the rate of drug absorption and excretion.
   • Epidemiology: For tracking disease spread under certain assumptions.
6. Environment Science
   • Population Dynamics: Quadratic equations can model certain population growth and decline scenarios.
   • Resource Management: Such as optimizing the harvesting rate for renewable resources.
7. Astronomy
   • Orbital Equations: Quadratic equations can describe certain types of planetary orbits.
8. Transportation
   • Route Optimization: Quadratic equations can help in optimizing the shortest or least time-consuming routes.
9. Games and Puzzles
   • Game Theory: Quadratic equations can model optimal strategies in certain zero-sum games.

Tips for Solving Quadratic Equations

• Always try to simplify the equation before solving it.
• Cross-verify your roots by substituting them back into the equation.
• Use computational tools for complex equations, but understand the underlying process.

These are just some examples, and the list can go on. Quadratic equations are an integral part of many aspects of science, engineering, and everyday life.

Conclusion

Quadratic equations are more than just an academic exercise; they find applications in various fields. Understanding how to solve these equations equips us with a tool that is widely applicable in solving real-world problems.

Liked this tool? Explore our other Math Tools.